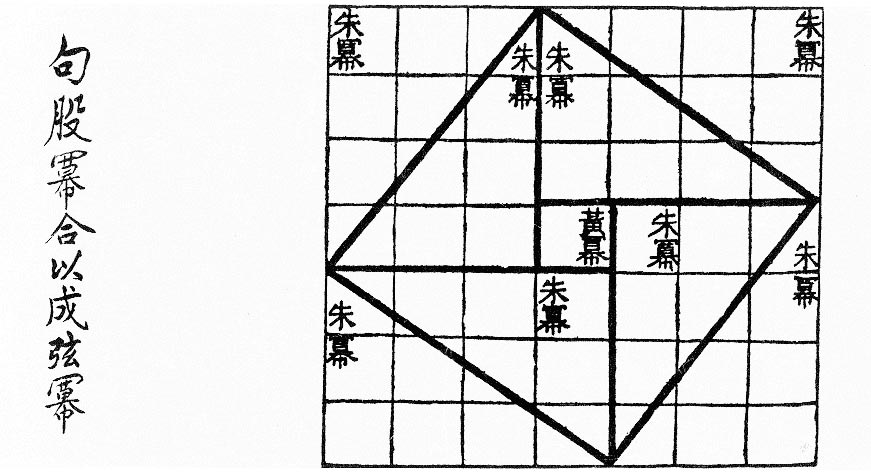
The Pythagorean theorem(translation is 毕达哥拉斯定理) in ancient Chinese mathematics is called 勾股定理.
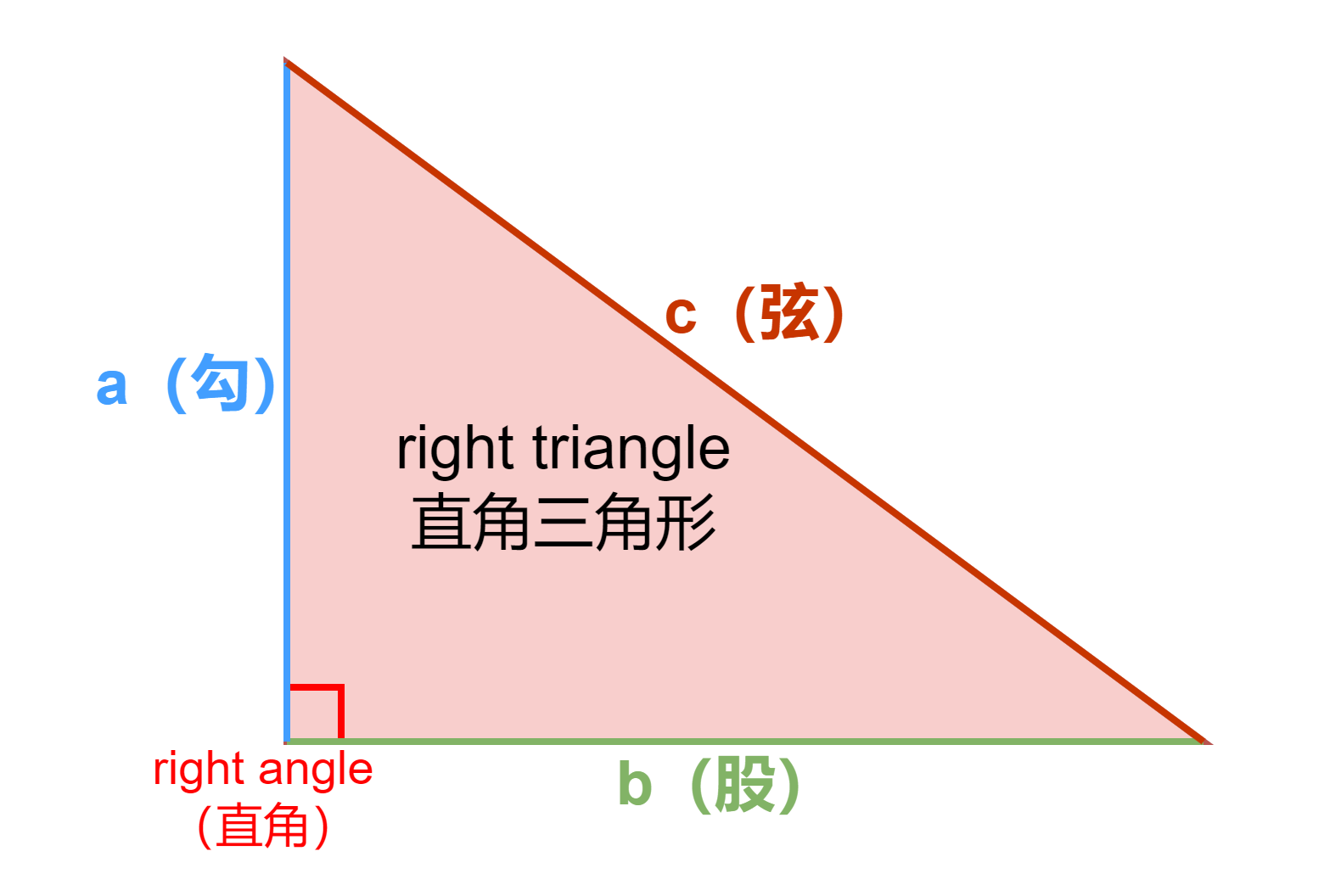
Typically, the mathematical expression we use to describe the Pythagorean Theorem is
a^2 + b^2 = c^2.
At the same time, we usually think of the hypotenuse of a right triangle as c, the short right side as a, and the long right side as b.
Therefore, a is shorter than b, and b is shorter than c.(a <= b, b < c)
Description of Pythagorean theorem in ancient Chinese mathematics
短面曰勾,长面曰股,相与结角曰弦。勾短其股,股短其弦。——《九章算术·勾股》
译文:
在直角三角形中,短的边称为勾,长的边称为股,与它们分别形成直角的边为弦。勾的长度比股短,股的长度比弦短。
In a right triangle, the short side is called 勾, the long side is called 股, and the side forming a right angle with them is 弦.
勾 is shorter than 股, and 股 is shorter than 弦.(勾 <= 股,股 < 弦)
Note that 冪 is the traditional Chinese character for 幂, and the 幂 mentioned below all refer to 冪.
勾股幂合以成弦幂
variant:
合勾股幂以成弦幂
以勾股幂合成弦幂
According to ancient grammar, there are two variations of this sentence, and I prefer the former.
勾 represents the short right-angled side, like a in the formula.股 represents the long right-angled side, like b in the formula.幂 means the product of two numbers multiplied together, here denoting self-multiplication, like the concept of square in modern math.
勾股幂 can be understood as the omitted expression of 勾幂和股幂.
合 means 结合, represents combine. It can be simply understood here as the sum of 勾幂 and 股幂.以成 means 用来形成, represents to become/form in English..
以 means 用来, represents the word to in English.成 means 变成 or 形成, represents become or form in English.
弦 represents the hypotenuse of a right triangle, like c in the formula.幂 as above.
Origin of 幂
about 巾(towel) :
巾,佩巾也。——《说文解字》
巾 is a pictographic character.
In the oracle bone script, its glyphs resemble a piece of fabric hanging down at both ends, indicating a textile for wiping, covering, wrapping, wearing, etc., much like modern towel.
about 幂(power) :
大巾谓之幂。——《小尔雅·广诂》
幂 is a phonetic word, and its sound side is 冥, indicating that the word and 冥 have similarities in pronunciation. Its shape is 巾, indicating that the word is related to the word 巾.
According to the structure of 幂 , it can be inferred that its original meaning is a towel used to cover things. It is then derived to mean to cover(覆盖,遮掩), and from that to mean area(面积).
about 冖 :
“冖,覆也,从一下垂也。” ——《说文解字》
Cover something with a square piece of cloth and let the four corners hang down to form the shape of an 冖.
Extending this meaning, anything that is square can also be called a 幂. By further extension, the area of a rectangle(矩形)1 or the product of two numbers2(especially the result of multiplying a number by itself [3]) is also called a power. This promotion began with Liu Hui(an ancient mathematician called 刘徽 in China).
"the product of two numbers" means 两数之积 in Chinese.
"multiplying a number by itself" means 自乘(一个数与自身相乘) in Chinese.
1
方田术曰:广纵步数相乘得积步。此积谓田幂。凡广纵相乘谓之幂。——《九章算术·方田》
译文
长方形田面积法则:宽与长的步数相乘得积步。(刘徽注:这个积称为田的幂。凡是长宽相乘就称为幂。)
The area rule of a rectangular field: multiply the number of steps of width and length to get the product of steps. (Liu Hui note: This product is called the 幂(power) of the field. Where length and width are multiplied together they are called 幂.)
步 (step) : In ancient times, each of the two feet crossed once called step, now refers to the distance between the two feet when walking.
2
又,勾自乘,以减弦自乘。其余,开方除之,即股。勾、股幂合以成弦幂,令去其一,则余在者皆可得而知之。——《九章算术·勾股》
译文:计算勾的自乘数,然后用弦的自乘数减去它,把余数作开方(根号)运算,结果就是股。
Calculate the self-multiplier of the 勾, then subtract it from the self-multiplier of the 弦, take the square root of the remainder, and the result is 股.
[3]
自乘之数曰幂。——《几何原本》by 徐光启, 利马窦
Now back to the question.
What does 朱幂 and 黄幂 mean in this ancient illustration of the Pythagorean Theorem?
about 朱幂 and 黄幂 :
朱幂 and 黄幂 here are names representing variables in the illustration.
朱 and 黄 each represent a color, and 幂 represents the area of a certain figure.
朱 means 赤色, indicate red.
朱,赤心木,松柏属。——《说文解字》
黄 here means yellow.
The eight 朱幂 in the illustration refer to the eight right triangles.
The 黄幂 refers to the middle square.
The 弦幂 I mentioned is a variable representing the large square around the perimeter.
The value of 朱幂 is the value of the area of the right triangle.
The value of 黄幂 is the value of the area of the small square in the middle.
The value of 弦幂 is the sum of four 朱幂 and one 黄幂.
In the picture I provided, the new variable 勾股差幂 refers to 黄幂.
勾股差幂 can better express the meaning of this small square.
Its value is the square of the result obtained by subtracting the value of 勾 from the value of 股.
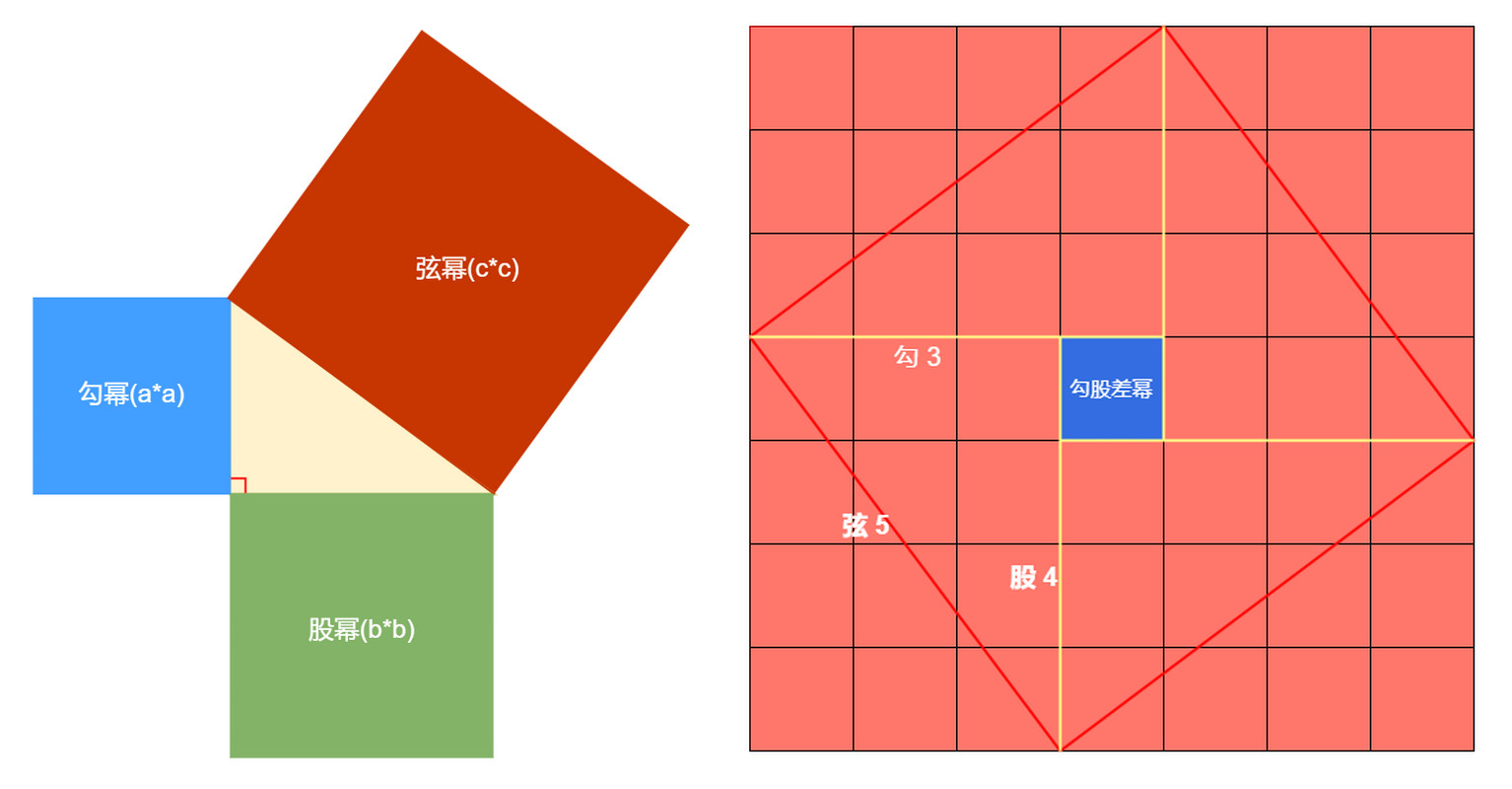
As can be seen from the illustration provided by the questioner:
朱幂 = 1/2 × (勾 × 股) = 1/2 × ab
黄幂 = (股 - 勾) × (股 - 勾) = (b - a)^2
弦幂 = 弦 × 弦 = c^2
The first kind of argument:
Four congruent right triangles form a square(弦幂) with side length c, and in the middle of the figure there is a small square(勾股差幂) with side length b – a.
because:
弦幂 = 4 × 朱幂 + 黄幂
thus:
c^2 = 1/2 × ab × 4 + (b - a)^2
c^2 = 2ab + a^2 + b^2 - 2ab
finally:
c^2 = a^2 + b^2
The second argument:
Then splice four identical congruent right triangles(朱幂) outside the square(弦幂) with side length c, and you will have a square with side length a + b, which can also prove the Pythagorean theorem.
because:
(勾股差幂 + 4 × 黄幂 ) + 4 × 黄幂 = (a + b)^2
弦幂 + 4 × 黄幂 = (a + b)^2
thus:
c^2 + 4 × (1/2 ab) = (a + b)^2
c^2 + 2 × ab = a^2 + b^2 + 2 × ab
finally:
c^2 = a^2 + b^2